In the self-organization process lead to spatial beam self-cleaning, one of the most important parameters to consider is the refractive index profile. For a parabolic transverse index distribution, the guided modes can be subdivided into groups with equally spaced propagation constants. The resulting Kerr-induced grating is at the origin of the phase matching process, which is able to couple modes that, at low powers, have different propagation constants, hence permitting to control the Kerr-beam self-cleaning dynamics.

Spatial beam self-cleaning has also been reported in active multimode optical fibers even in the case of a non-parabolic refractive-index profile. However, our experiments show that a deviation from a perfect parabolic shape increases the power threshold for beam cleaning, and consequently reduces the amount of power transferred to the “cleaned” mode. Therefore, besides the choice of the input conditions, the principal ingredients for beam self-cleaning are the fiber characteristics, including the refractive index profile, the core diameter and the nonlinear refractive index of the glass. In this context, fiber technology can play a pivotal role, by permitting to design complex guiding profiles with longitudinal and/or transverse structuration of dopants that can in turn enable the control of velocity differences among the guided modes, hence the efficiency of energy exchange among them. Unfortunately, the control of the refractive index profile and active ions currently remains a challenging task, which is not yet completely mastered.

In 1995 Ballato et al. used a new fiber fabrication method called “powder in tube” with the aim of increasing rare-earth concentration in optical fiber lasers [1]. This method was based on the melting of a powder composition in a capillary tube during the drawing process. It offers a large degree of freedom in the fiber design, thus allowing one to realize symmetric or asymmetric fibers composed of several different materials. Moreover, this method can be easily combined with the stack-and-draw process. With the powder in tube method, the mutual diffusion of different chemical compositions between the core and the cladding can contribute to shaping the refractive index profile and the doping concentration. Interestingly, such a technique may permit the fabrication of very large core active fibers with a controlled repartition of rare-earth ions. The method has been further improved by implementing a modified powder-in-tube technique (MPIT), which consists of a consolidation process taking place in the preparation of the preform, either under vacuum or pressurized gas, and where particular properties of the fiber were achieved [2].

We demonstrate that by using the MPIT method, it is possible to draw a multimode lanthanum aluminum silicate fiber (MLASF) with quasi-parabolic refractive index profile, exhibiting losses that are low enough to permit observing nonlinear effects such as the spatial self-cleaning.

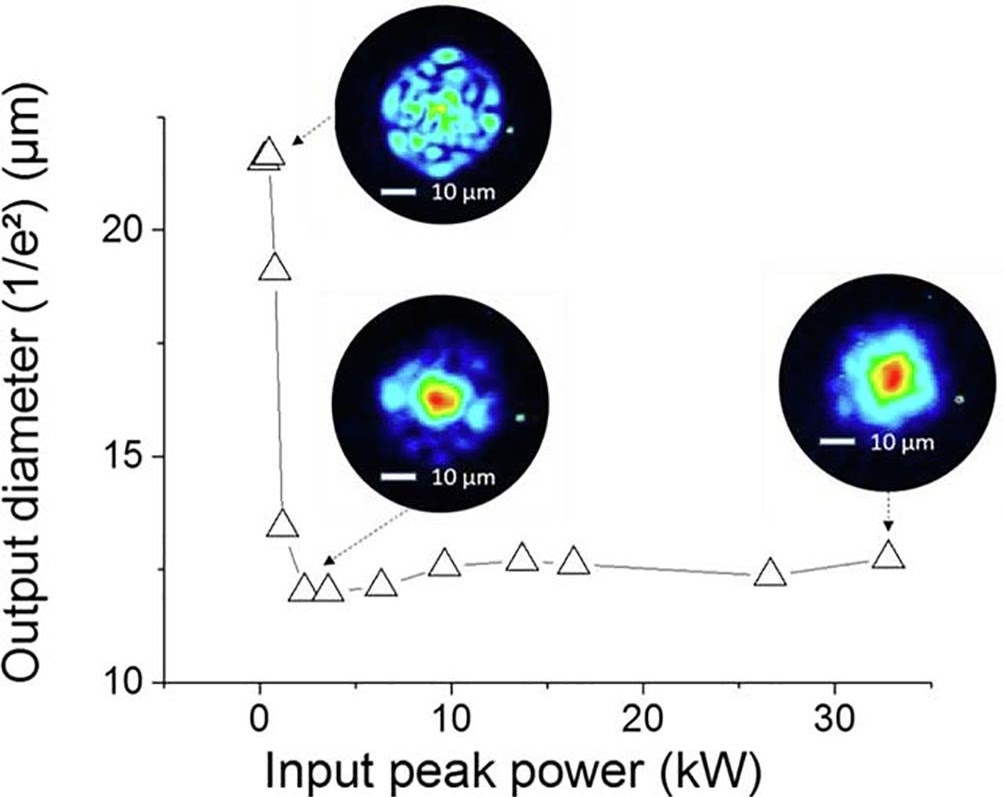
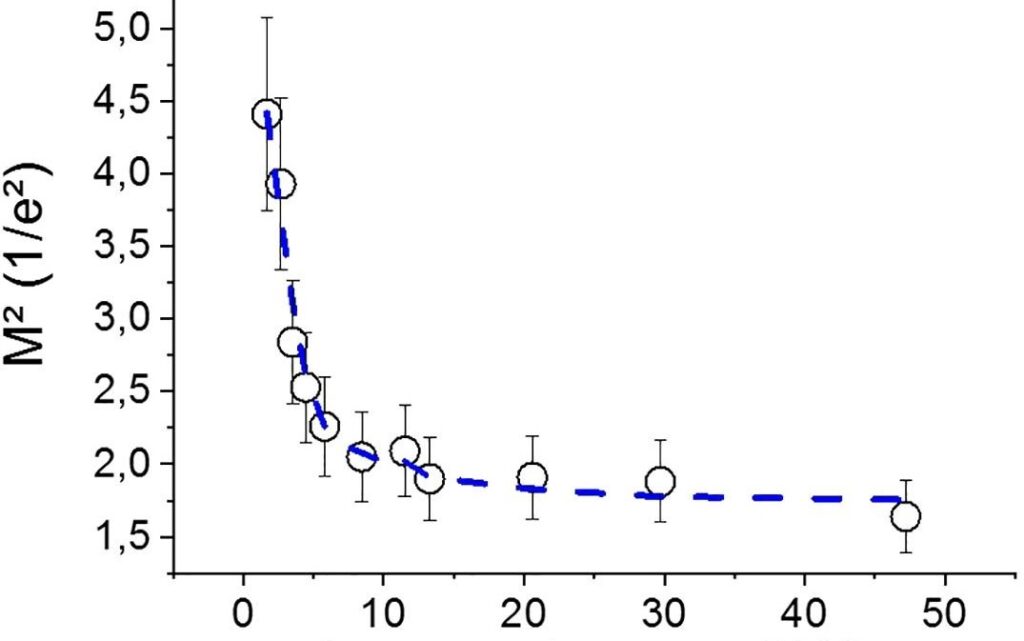
We characterized the output spatial beam shape as a function of the power injected into the MLASF. As can be seen from the insets of Fig. 1, for low values of input peak power, the output beam exhibited a speckled pattern, owing to the simultaneous excitation of many transverse modes propagating with their own phase velocity. Correspondingly, Fig. 2 shows that the beam quality at the output end of the fiber appeared to be quite poor.

However, by increasing the input peak power and by keeping unchanged the position of the input beam, we observed a gradual change in the energy distribution that was progressively concentrated at the center of the fiber core. When we reached the maximum value of 32 kW peak power coupled in the multimode fiber, the diameter of the output beam reached a value close to 12 µm (at 1/e2), and the M2 coefficient evolved from 4.5 down to 1.6 (see Fig. 2). The nonlinear beam reshaping observed at the fiber end entails a significant brightness enhancement of the output beam.

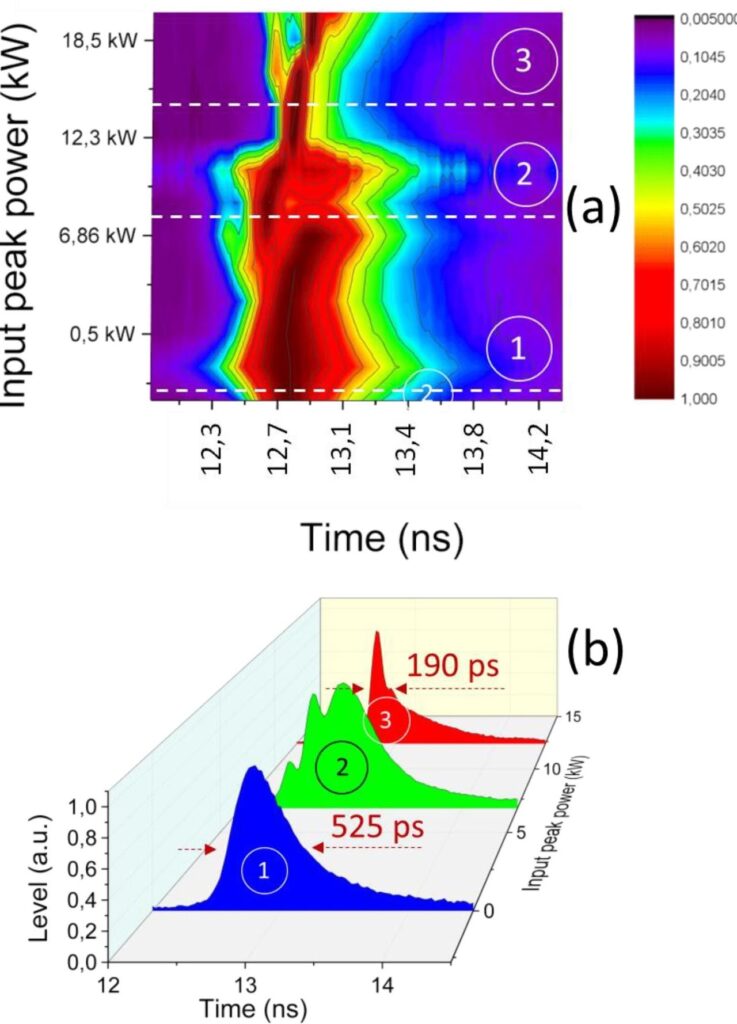
We also measured the signature of Kerr beam self-cleaning in the time domain. To do so, we magnified the output beam, so that only the central part was detected by the small effective area of an ultrafast photodetector. We recorded the temporal pulse profile at the center of the output beam, which mainly contains the fundamental transverse mode, for increasing input power values. Our results are summarized in Fig. 3. The energy exchange among modes is mainly driven by the instantaneous peak power carried in each of them. Therefore, an initial bell-shaped pulse is drastically modulated in time upon propagation, with the appearance of several sharp peaks for different values of the instantaneous intensity.

1. John Ballato, Elias Snitzer, Fabrication of fibers with high rare-earth concentrations for Faraday isolator application, Appl. Optics, 34 (30) (1995), pp. 6848-6854
2. Jean-Louis Auguste, Georges Humbert, Stéphanie Leparmentier, Maryna Kudinova, Pierre-Olivier Martin, Gaëlle Delaizir, Kay Schuster, Doris Litzkendorf, Modified powder-in-tube technique based on the consolidation processing of powder materials for fabricating specialty optical fibers, Materials, 7 (2014), pp. 6045-6063